Secrets of Mental Math by Arthur Benjamin (namjoon book recommendations TXT) 📖

by 11 if and only if you arrive at either 0 or a multiple of 11 when you alternately subtract and add the digits of a number. For instance, 73,958 is not divisible by 11 since 7−3 + 9 − 5 + 8 = 16. However, the numbers 8,492 and 73,194 are multiples of 11, since 8 − 4 + 9 − 2 = 11 and 7 − 3 + 1 − 9 + 4 = 0. The reason this works is based, like the rule for 3s and 9s, on the fact that the numbers 1, 100, 10,000, and 1,000,000 are 1 more than a multiple of 11, whereas the numbers 10, 1,000, 100,000, and so on are 1 less than a multiple of 11.

Testing for divisibility by 7 is a bit trickier. If you add or subtract a number that is a multiple of 7 to the number you are testing, and the resulting number is a multiple of 7, then the test is positive. I always choose to add or subtract a multiple of 7 so that the resulting sum or difference ends in 0. For example, to test the number 5292, I subtract 42 (a multiple of 7) to obtain 5250. Next, I get rid of the 0 at the end (since dividing by ten does not affect divisibility by seven), leaving me with 525. Then I repeat the process by adding 35 (a multiple of 7), which gives me 560. When I delete the 0, I’m left with 56, which I know to be a multiple of 7. Therefore, the original number 5292 is divisible by 7.

This method works not only for 7s, but also for any odd number that doesn’t end in 5. For example, to test whether 8792 is divisible by 13, subtract 4 × 13 = 52 from 8792, to arrive at 8740. Dropping the 0 results in 874. Then add 2 × 13 = 26 to arrive at 900. Dropping the two 0s leaves you with 9, which is clearly not a multiple of 13. Therefore, 8792 is not a multiple of 13.

In this final set of exercises, be especially careful when you test for divisibility by 7 and 17. The rest should be easy for you.

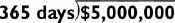
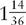
FRACTIONS

If you can manipulate whole numbers, then doing arithmetic with fractions is almost as easy. In this section, we review the basic methods for adding, subtracting, multiplying, dividing, and simplifying fractions. Those already familiar with fractions can skip this section with no loss of continuity.

Multiplying Fractions

To multiply two fractions, simply multiply the top numbers (called the numerators), then multiply the bottom numbers (called the denominators). For example,

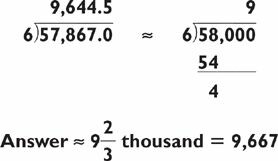
What could be simpler! Try these exercises before going further.

Dividing Fractions

Dividing fractions is just as easy as multiplying fractions. There’s just one extra step. First, turn the second fraction upside down (this is called the reciprocal) then multiply. For instance, the reciprocal of ⅘ is 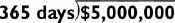. Therefore,

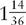
Now it’s your turn. Divide these fractions.

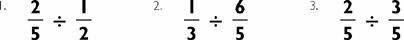
Simplifying Fractions

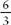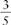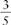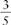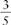Fractions can be thought of as little division problems. For instance, is the same as 6 ÷ 3 = 2. The fraction is the same as 1 ÷ 4 (which is .25 in decimal form). Now we know that when we multiply any number by 1, the number stays the same. For example, = × 1. But if we replace 1 with , we get = × 1 = 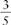× = 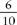. Hence, 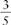= 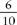Likewise, if we replace 1 with 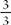, we get 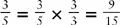. In other words, if we multiply the numerator and denominator by the same number, we get a fraction that is equal to the first fraction.

For another example,

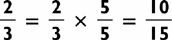
It is also true that if we divide the numerator and denominator by the same number, then we get a fraction that is equal to the first one.

For instance,

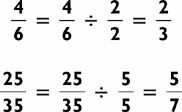
This is called simplifying the fraction.

For the fractions below, can you find an equal fraction whose denominator is 12?

Simplify these fractions.

Adding Fractions

The easy case is that of equal denominators. If the denominators are equal, then we add the numerators and keep the same denominators.

For instance,

Sometimes we can simplify our answer. For instance,

The trickier case: unequal denominators. When the denominators are not equal, then we replace our fractions with fractions where the denominators are equal.

For instance, to add

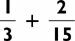
we notice that

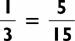
Therefore,

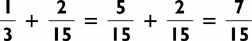
To add

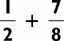
we notice that

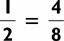
So,

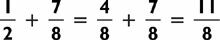
To add

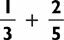
we see that

So,

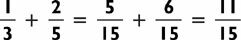
Subtracting Fractions

Subtracting fractions works very much like adding them. We have illustrated with examples and provided exercises for you to do.

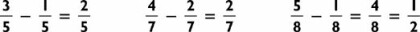
Chapter 5 Good Enough: The Art of “Guesstimation”

Chapter 5

Good Enough: The Art
of “Guesstimation”

So far you’ve been perfecting the mental techniques necessary to figure out the exact answers to math problems. Often, however, all you’ll want is a ballpark estimate. Say you’re getting quotes from different lenders on refinancing your home. All you really need at this information-gathering stage is a ballpark estimate of what your monthly payments will be. Or say you’re settling a restaurant bill with a group of friends and you don’t want to figure each person’s bill to the penny. The guesstimation methods described in this chapter will make both these tasks—and many more just like them—much easier. Addition, subtraction, division, and multiplication all lend themselves to guesstimation. As usual, you’ll do your computations from left to right.

ADDITION GUESSTIMATION

Guesstimation is a good way to make your life easier when the numbers of a problem are too long to remember. The trick is to round the original numbers up or down:

George Parker Bidder: The Calculating Engineer

The British have had their share of lightning calculators, and the mental performances of George Parker Bidder (1806–1878), born in Devonshire, were as impressive as any. Like most lightning calculators, Bidder began to try his hand (and mind) at mental arithmetic as a young lad. Learning to count, add, subtract, multiply, and divide by playing with marbles, Bidder went on tour with his father at age nine.

Almost no question was too difficult for him to handle. “If the moon is 123,256 miles from the earth and sound travels four miles a minute, how long would it take for sound to travel from the earth to the moon?” The young Bidder, his face wrinkled in thought for nearly a minute, replied, “Twenty-one days, nine hours, thirty-four minutes.” (We know now that the distance is closer to 240,000 miles and sound cannot travel through the vacuum of space.) At age ten, Bidder mentally computed the square root of 119,550,669,121 as 345,761 in a mere thirty seconds. In 1818, Bidder and the American lightning calculator Zerah Colburn were paired in a mental calculating duel in which Bidder, apparently, “outnumbered” Colburn.

Riding on his fame, George Bidder entered the University of Edinburgh and went on to become one of the more respected engineers in England. In parliamentary debates over railroad conflicts, Bidder was frequently called as a witness, which made the opposition shudder; as one said, “Nature had endowed him with particular qualities that did not place his opponents on a fair footing.” Unlike Colburn, who retired as a lightning calculator at age twenty, Bidder kept it up for his entire life. As late as 1878, in fact, just before his death, Bidder calculated the number of vibrations of light striking the eye in one second, based on the fact that there are 36,918 waves of red light per inch, and light travels at approximately 190,000 miles per second.

Notice that we rounded the first number down to the nearest thousand and the second number up. Since the exact answer is 14,186, our relative error is small.

If you want to be more exact, instead of rounding off to the nearest thousand, round off to the nearest hundred:

The answer is only 14 off from the exact answer, an error of less than .1%. This is what I call a good guesstimation!

Try a five-digit addition problem, rounding to the nearest hundred:

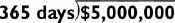
By rounding to the nearest hundred, our answer will always be off by less than 100. If the answer is larger than 10,000, your guesstimate will be within 1% of the exact answer.

Now let’s try something wild:

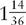
If you round to the nearest million, you get an answer of 31 million, off by roughly 285,000. Not bad, but you can do better by rounding to the nearest hundred thousand, as we’ve shown in the right-hand column. Again your guesstimate will be within 1% of the precise answer. If you can compute these smaller problems exactly, you can guesstimate the answer to any addition problem.

Guesstimating at the Supermarket

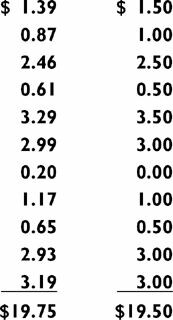
Let’s try a real-world example. Have you ever gone to the store and wondered what the total is going to be before the cashier rings it up? For estimating the total, my technique is to round the prices to the nearest 50¢. For example, while the cashier is adding the numbers shown below on the left, I mentally add the numbers shown on the right:

My final figure is usually within a dollar of the exact total.

SUBTRACTION GUESSTIMATION

The way to guesstimate the answers to subtraction problems is the same—you round to the nearest thousand or hundreds digit, preferably the latter:

You can see that rounding to the nearest thousand leaves you with an answer quite a bit off the mark. By rounding to the second digit (hundreds, in the example), your answer will usually be within 3% of the exact answer. For this problem, your answer is off by only 52, a relative error of 2%. If you round to the third digit, the relative error will usually be below 1%. For instance:

By rounding the numbers to the third digit rather than to the second digit, you improve the accuracy of the estimate by a significant amount.

DIVISION GUESSTIMATION

The first, and most important, step in guesstimating the answer to a division problem is to determine the magnitude of the answer:

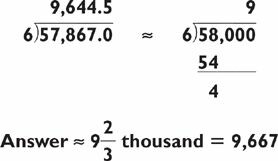
The next step is to round off the larger numbers to the nearest thousand and change the 57,867 to 58,000. Dividing 6 into 58 gives you 9 with a remainder. But the most important component in this problem is where to place the 9.

For example, multiplying 6 × 90 yields 540, while multiplying 6 × 900 yields 5,400, both of which are too small. But 6 × 9,000 = 54,000, which is pretty close to the answer. This tells you the answer is 9,000 and something. You can estimate just what that something is by first subtracting 58 − 54 = 4. At this point you could bring down the 0 and divide 6 into 40, and so forth. But if you’re on your toes, you’ll realize that dividing 6 into 4 gives you .667. Since you know the answer is 9,000 something, you’re now in a position to guess 9,667. In fact, the actual answer is 9,645—darn close!

Division on this level is simple. But what about large division problems? Let’s say we want to compute, just for fun, the amount of money a professional athlete earns a day if he makes $5,000,000