Secrets of Mental Math by Arthur Benjamin (namjoon book recommendations TXT) 📖

was thrilled. Thoughts of “Benjamin’s Theorem” popped into my head. I actually believed I had discovered something new. When I finally ran across this method a few years later in a book by Martin Gardner on recreational math, Mathematical Carnival (1965), it ruined my day! Still, the fact that I had discovered it for myself was very exciting to me.

You, too, can impress your friends (or teachers) with some fairly amazing mental multiplication. At the end of the last chapter you learned how to multiply a two-digit number by itself. In this chapter you will learn how to multiply two different two-digit numbers, a challenging yet more creative task. You will then try your hand—or, more accurately, your brain—at three-digit squares. You do not have to know how to do 2-by-2 multiplication problems to tackle three-digit squares, so you can learn either skill first.

2-BY-2 MULTIPLICATION PROBLEMS

When squaring two-digit numbers, the method is always the same. When multiplying two-digit numbers, however, you can use lots of different methods to arrive at the same answer. For me, this is where the fun begins.

The first method you will learn is the “addition method,” which can be used to solve all 2-by-2 multiplication problems.

The Addition Method

To use the addition method to multiply any two two-digit numbers, all you need to do is perform two 2-by-1 multiplication problems and add the results together. For example:

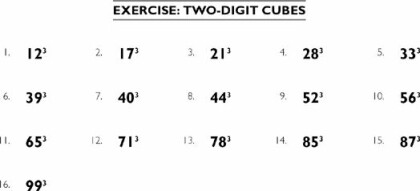
Here you break up 42 into 40 and 2, two numbers that are easy to multiply. Then you multiply 40 × 46, which is just 4 × 46 with a 0 attached, or 1840. Then you multiply 2 × 46 = 92. Finally, you add 1840 + 92 = 1932, as diagrammed above.

Here’s another way to do the same problem:

The catch here is that multiplying 6 × 42 is harder to do than multiplying 2 × 46, as in the first problem. Moreover, adding 1680 + 252 is more difficult than adding 1840 + 92. So how do you decide which number to break up? I try to choose the number that will produce the easier addition problem. In most cases—but not all—you will want to break up the number with the smaller last digit because it usually produces a smaller second number for you to add.

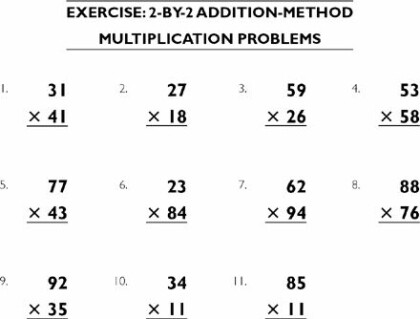
Now try your hand at the following problems:

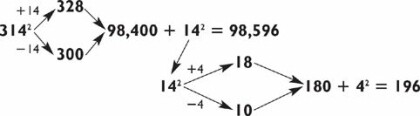
The last problem illustrates why numbers that end in 1 are especially attractive to break up. If both numbers end in the same digit, you should break up the larger number as illustrated below:

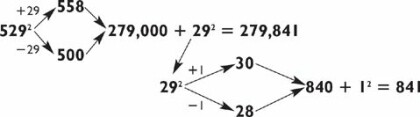
If one number is much larger than the other, it often pays to break up the larger number, even if it has a larger last digit. You will see what I mean when you try the following problem two different ways:

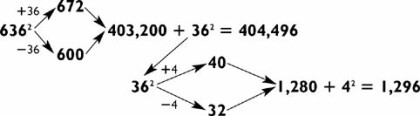
Did you find the first method to be faster than the second? I did.

Here’s another exception to the rule of breaking up the number with the smaller last digit. When you multiply a number in the fifties by an even number, you’ll want to break up the number in the fifties:

The last digit of the number 84 is smaller than the last digit of 59, but if you break up 59, your product will be a multiple of 100, just as 4200 is, in the example above. This makes the subsequent addition problem much easier.

Now try an easy problem of a different sort:

Though the calculation above is pretty simple, there is an even easier and faster way to multiply any two-digit number by 11. This is mathemagics at its best: you won’t believe your eyes when you see it (unless you remember it from Chapter 0)!

Here’s how it works. Suppose you have a two-digit number whose digits add up to 9 or less. To multiply this number by 11, merely add the two digits together and insert the total between the original two digits. For example, to do 42 × 11, first do 4 + 2 = 6. If you place the 6 between the 4 and the 2, you get 462, the answer to the problem!

Try 54 × 11 by this method.

What could be simpler? All you had to do was place the 9 between the 5 and the 4 to give you the final answer of 594.

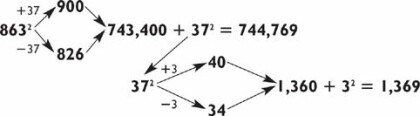
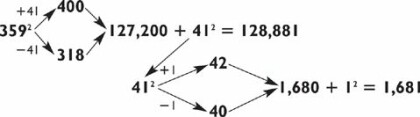
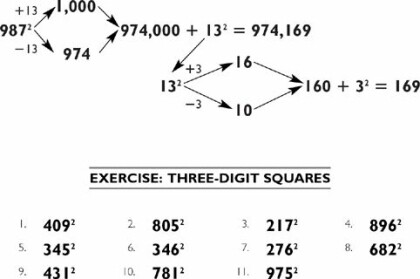
You may wonder what happens when the two numbers add up to a number larger than 9. In such cases, increase the tens digit by 1, then insert the last digit of the sum between the two numbers, as before. For example, when multiplying 76 × 11, 7 + 6 = 13, you increase the 7 in 76 to 8, and then insert the 3 between the 8 and the 6, giving you 836, the final answer. See the diagrammed answer below:

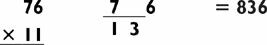
Your turn. Try 68 × 11.

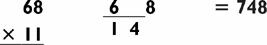
Once you get the hang of this trick, you will never multiply by 11 any other way. Try a few more problems and then check your answers at the back of the book.

Returning to the addition method, the next problem is a real challenge the first time you try it. Try solving 89 × 72 in your head, looking back at the problem if necessary. If you have to start over a couple of times, that’s okay.

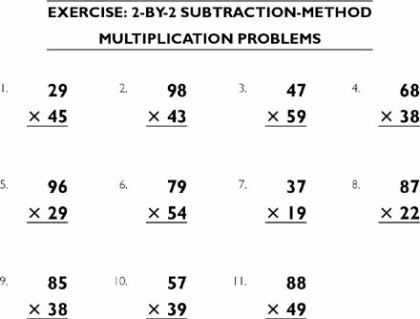
If you got the right answer the first or second time, pat yourself on the back. The 2-by-2 multiplication problems really do not get any tougher than this. If you did not get the answer right away, don’t worry. In the next two sections, I’ll teach you some much easier strategies for dealing with problems like this. But before you read on, practice the addition method on the following multiplication problems.

The Subtraction Method

The subtraction method really comes in handy when one of the numbers you want to multiply ends in 8 or 9. The following problem illustrates what I mean:

Although most people find addition easier than subtraction, it is usually easier to subtract a small number than to add a big number. (If we had done this problem by the addition method, we would have added 850 + 153 = 1003.)

Now let’s do the challenging problem from the end of the last section:

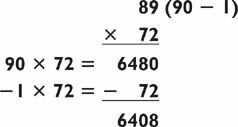
Wasn’t that a whole lot easier? Now, here’s a problem where one number ends in 8:

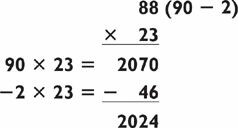
In this case you should treat 88 as 90 − 2, then multiply 90 × 23 = 2070. But you multiplied by too much. How much? By 2 × 23, or 46 too much. So subtract 46 from 2070 to arrive at 2024, the final answer.

I want to emphasize here that it is important to work out these problems in your head and not simply look to see how we did it in the diagram. Go through them and say the steps to yourself or even out loud to reinforce your thoughts.

Not only do I use the subtraction method with numbers that end in 8 or 9, but also for numbers in the high 90s because 100 is such a convenient number to multiply. For example, if someone asked me to multiply 96 × 73, I would immediately round up 96 to 100:

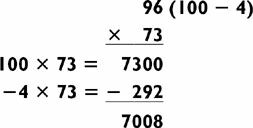
When the subtraction component of a multiplication problem requires you to borrow a number, using complements (as we learned in Chapter 1) can help you arrive at the answer more quickly. You’ll see what I mean as you work your way through the problems below. For example, subtract 340 − 78. We know the answer will be in the 200s. The difference between 40 and 78 is 38. Now take the complement of 38 to get 62. And that’s the answer, 262!

Now let’s try another problem:

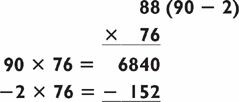
There are two ways to perform the subtraction component of this problem. The “long” way subtracts 200 and adds back 48:

The short way is to realize that the answer will be 66 hundred and something. To determine something, we subtract 52 − 40 = 12 and then find the complement of 12, which is 88. Hence the answer is 6688.

Try this one.

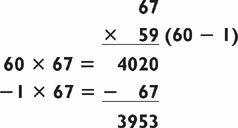
Again, you can see that the answer will be 3900 and something. Because 67 − 20 = 47, the complement 53 means the answer is 3953.

As you may have realized, you can use this method with any subtraction problem that requires you to borrow a number, not just those that are part of a multiplication problem. All of this is further proof, if you need it, that complements are a very powerful tool in mathemagics. Master this technique and, pretty soon, people will be complimenting you!

The Factoring Method

The factoring method is my favorite method of multiplying two-digit numbers since it involves no addition or subtraction at all. You use it when one of the numbers in a two-digit multiplication problem can be factored into one-digit numbers.

To factor a number means to break it down into one-digit numbers that, when multiplied together, give the original number. For example, the number 24 can be factored into 8 × 3 or 6 × 4. (It can also be factored into 12 × 2, but we prefer to use only single-digit factors.)

Here are some other examples of factored numbers:

42 = 7 × 6
63 = 9 × 7
84 = 7×6×2 or 7×4×3

To see how factoring makes multiplication easier, consider the following problem:

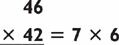
Previously we solved this problem by multiplying 46 × 40 and 46 × 2 and adding the products together. To use the factoring method, treat 42 as 7 × 6 and begin by multiplying 46 × 7, which is 322. Then multiply 322 × 6 for the final answer of 1932. You already know how to do 2-by-1 and 3-by-1 multiplication problems, so this should not be too hard:

46 × 42 = 46 × (7 × 6) = (46 × 7) × 6 = 322 × 6 = 1932

Of course, this problem could also have been solved by reversing the factors of 42:

46 × 42 = 46 × (6 × 7) = (46 × 6) × 7 = 276 × 7 = 1932

In this case, it is easier to multiply 322 × 6 than it is to multiply 276 × 7. In most cases, I like to use the larger factor in solving the initial 2-by-1 problem and to reserve the smaller factor for the 3-by-1 component of the problem.

Factoring results in a 2-by-2 multiplication problem being simplified to an easier 3-by-1 (or sometimes 2-by-1) multiplication problem. The advantage of the factoring method in mental calculation is you do not have to hold much in memory. Let’s look at another example, 75 × 63:

75 × 63 = 75 × (9 × 7) = (75 × 9) × 7 = 675 × 7 = 4725

As before, you simplify this 2-by-2 problem by factoring 63 into 9 × 7 and then multiplying 75 by these factors. (By the way, the reason we can shift parentheses in the second step is the associative law of multiplication.)

63 × 75 = 63 × (5 × 5 × 3) = (63 × 5) × 5 × 3 = 315 × 5 × 3 = 1575 × 3 = 4725

Try the following problem for practice:

57 × 24 = 57 × 8 × 3 = 456 × 3 = 1368

You could have factored 24 as 6 × 4 for another easy computation:

57 × 24 = 57 × 6 × 4 = 342 × 4 = 1368

Compare this approach with the addition method:

With the addition method, you have to perform two 2-by-1 problems and then add. With the factoring method, you have just two multiplication problems: a 2-by-1 and a 3-by-1, and then you are done. The factoring method is usually easier on your memory.

Remember that challenging multiplication problem earlier in this chapter? Here it is again:

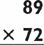
We tackled that problem easily enough with the subtraction method, but factoring works even faster:

89 × 72 = 89 × 9 × 8 = 801 × 8 = 6408

The problem is especially easy because of the 0